*
AFTERMATH
In anticipation of the criticism that I am making an irresponsible attack on the foundations of mathematics, offering nothing in its place, I would like to put my cards on the table. Let the reader assess for himself whether my critique has a sound basis, and whether it is not therefore capable of reinforcing mathematics in areas currently shaky - and perhaps, by directing our attention to the meaning of its formalisms, of making mathematics even better.
Essentially what I have done is to point out certain distinctions - between the degree of an equation, the order of a derivative, the different types of number and so on. My perception of these distinctions was made possible when, having been exposed to the wisdom of ancient teachings - the pre-Greek, Egyptian, American Indian, biblical and others - I realized that the gods of the four directions, the four elements, the four sons of Horus and other "fours" to which these teachings refer constituted a profound and comprehensive analytical tool, a classification comparable to what was initiated but not completed in modern times by Bertrand Russell's "logical types."
(Russell's logical types were incomplete because he perceived only two: "The class of elephants," he said, "is not an elephant.")
These types or categories were rediscovered by Aristotle in his four causes. They have been in part revived in the coordinate system - the complex coordinate system especially - but have been deprived of much of their content by the restriction of their use to measure.
The fourfold system emerges again in the four functions of Jung - intuition, emotion, intellect and sensation - which are easily recognized distinctions but which Jung elevated into a formalism by emphasizing their mutual opposition and complementarity.
Another example is in Newton's calculus, specifically the derivatives of position with respect to time, which we showed earlier to be a four operator. As we said, these are known through different faculties: we sense position, compute velocity, feel acceleration and do control.
In geometry we have point, line, plane and solid; in mathematics we have four kinds of transformation of coordinates - rotation, scale, inversion and linear translation. (Inversion is x' = 1/x, as occurs when the points outside a unit circle are put in correspondence with the points inside the unit circle; this puts infinity at the center.) Also in mathematics we have quadratic equations, so called because they involve four roots of unity, displayed on complex coordinates (see p. 26). Of course, there are cubic, quintic, and other power of equations, but the cubic is solved by reducing it to quadratic form. Again the unit circle can be divided equally into three, five or six parts (or multiples thereof) by the use of square roots, that is, by solving quadratic equations.
So what? This merely restates what is already obvious
to mathematics. Why is this important?To show more general significance I have to go back to the four elements, or "the gods of the four directions," to each of which were assigned a different meaning, whereas
mathematics assumes directions to be isotropic, that is, the directions of space are of the same nature.The difference between the four elements - or between the four causes, or the four functions - is crucial; it constitutes their contribution. Thus Aristotle's final cause is the purpose, or function (of, say, a chair or a machine); the material cause is the material of which it is made; the formal cause is the plan or blueprint; and the efficient cause (from facio, to make) is the work of making it.
At the risk of some confusion, best noted at the outset, the material cause can also be the need for something, need being distinguished from purpose in that it is phenomenal, that is, changing, whereas the purpose is noumenal. Thus the need for food diminishes when food is supplied, where as the purpose of food doesn't change.
The four elements, fire, water, air and earth, would
seem to be a reference to the four states of matter plasma, liquid, gaseous and solid - which is how they were interpreted by Greek philosophers. But this interpretation is too literal; their true meaning is much closer, indeed identical, to the four causes, showing that Aristotle could not have understood the four elements or he would not have invented the four causes. Be that as it may, we can get a grasp of their meaning from their common usage in our language. Thus "to fire" is to start, as to ignite a fire, to spark an endeavor; it can also mean to project or throw out, as in projecting a goal, or firing a person from a job.Fire, as the initiating cause, is thus best translated as purpose.
Water is difficult, but through its connection with feeling as well as the fact that water is substantial but devoid of fixed form, it corresponds to material cause and to emotion and need. Thus, Eve introduces desire into cosmology
and becomes "the mother of all living. " Another of these prerational or preconceptual correlations is that matter has the same root, mater, as "mother," and again, we say something "matters" when we mean that we value it. Water then is best translated as value.Air as an element stands for the mental or conceptual aspect of things. Thus we "air" our opinion, or put it on the air, that is, communicate and make known. Air is spatial relationship, concept, and is best translated as form.
Earth is the combination of substance (water) and form (air), or "formed substance;" when used as a verb, to earth means to put into practice, make practical. It represents the tangible, objective aspect and can best be translated as object.
The correlation of the elements with the four causes is obvious, and with the four functions is fairly so - fire with intuition, water with emotion (which according to Jung evaluates), air with intellect, and earth with sensation. But I want to show the relevance to mathematics of the four elements as categories of meaning, and this provokes the charge that the elements are of anthropomorphic origin and therefore not suitable for the abstract science of pure thought. While it could well be argued that "pure thought" is but one aspect of human functioning (air), I will sidestep this issue and translate the four elements into dimensionality and thus eliminate their "anthropomorphic contamination." We can then show their relevance to mathematics.
Fire is centrifugal, exploding out from a point like a bullet fired from a gun; it has no constraint. The choice of direction is not predetermined; it is the option that starts any process. We can therefore say it is zerodimensional, like a point. The "point" is also synonomous with "purpose."
Water is energy, desire, need, or the charge that propels the bullet. Its measure is one-dimensional (the amount of energy is denoted by one number), like the line, one constraint.
Air describes the space occupied and hence form; it defines, limits, and must have two axes. It depicts proportion, the relationship structure, as does a blueprint, and is two-dimensional, like the plane.
Earth, formed objects, has volume or three dimensions, like a solid.
In the above, dimensions are thought of as constraints.
(Three specifications are necessary to locate a point in three-space.) Of course, in mathematics there can be as many dimensions as we please, but in such usage dimensions are rather variables and the notion of four categories is not applicable.
A richer example of the four distinctions, which gets closer to the ontological implications of measurement, was introduced in a previous essay, "Constraint and Freedom" (1980). There I showed that the most generally used coordinate system, the Cartesian, with its three axes x, y, and z, while ideal for describing objects - as in architectural or mechanical drawings - does not portray important aspects such as scale and orientation (except with reference to other objects). In manufacture, orientation is provided by what is called the next assembly drawing, which shows how the part is fitted into the rest of the machine. Scale is provided in drawings and in maps by a line on the same sheet of paper, or by a statement "ten miles to the inch," or the like, which is a reference to a physical object - either the mark on the map or the standard inch.
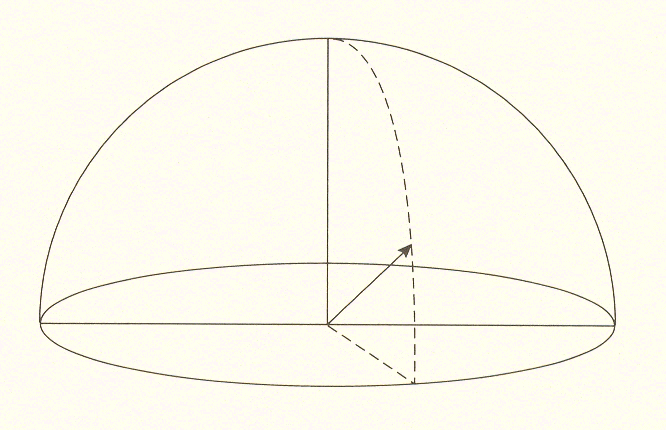
A more inclusive method, the spherical coordinate systems, has its origin at the observer, and hence does provide scale and orientation:
It maps, say, the stars in terms of latitude and longitude (measures analogous to the two dimensions of a map), and also gives scale in the measure R, or "distance away" from the origin. Orientation is provided in that the measurements of longitude and latitude are from the same origin for all maps. This makes it possible for maps of a small sector of the heavens or of the earth's surface to be located in the whole picture - the whole surface of the earth.
Of course, the fact that the map is not the territory still holds for spherical coordinates. The relationship between points on the map, together with scale that requires a physical object, the orientation, which for a map is a compass, and the territory, are the four different categories described above, which can be properly thought of as four levels.
The mathematician may continue to object that these references and examples are of lower dignity than mathematics itself, which he may still regard as operating in an ideal world. But my argument in the present work is that such reality conditions as the three-dimensionality of space and the asymmetry of time are not mere contingencies of this particular universe, but clues to a theoretical science that belongs in the province of mathematic as queen of the sciences. Again, when we can show that the extraction of roots can be interpreted as creating space, we cannot put geometry aside as inferior; it literally has its "roots" in mathematics.
But let me get back to the four levels through another approach. While the enthusiasm for logicizing mathematics has perhaps had its demise, it has never been replaced, even though Goedel developed a formalism by which he was able to prove that logic was incomplete. I must say that I am disappointed that more has not been developed from this start. Instead, 'Goedel's demonstration is preserved like the leaning tower of Pisa, as a tourist attraction, something interesting in an otherwise tedious subject.
Goedel's proof applies to logic only if logic is extended to include arithmetic. In order to include arithmetic the Peano axioms are added to the axioms of logic. In a previous paper, "Postulates and Logic" (1962), I distinguished postulates from definitions, requiring of the former that they must have a meaningful negation. I pointed out
that the postulates of propositional logic as given by Church, when stripped of what are essentially definitions, reduce to two: that every proposition has an antecedent and that every proposition is general. We then add the Peano axioms to extend logic to include arithmetic. These axioms set forth the undefined concepts number, successor and zero.- Zero is a number and zero has no predecessor.
- Zero has a successor (one) and the successor of zero has a successor (two) and so on.
But what have we done? By the admission of zero, defined as having no predecessor, we have violated the axiom of logic that every proposition has an antecedent. We have also violated the axiom of generality in that zero is unique; it is the only number that has no predecessor, and each other number is unique because it is the only (immediate) successor of the previous number. No wonder logic breaks down when we admit entities in violation of its two postulates.
So it is not that logic per se is at fault. The cause is rather in a mistaken ideology, a kind of wedding with no
consummation between logic and mathematics. I don't think mathematics was aware that it had been married to logic, and the result of the attempt to logicize mathematics, symbolic logic, has been used as a substitute for meaning rather than to express it.The trouble lies in trying to extend logic where it does not, by its own definition, apply. The implication is that there is such a domain to which logic does not apply; in fact, there are precisely three such domains.
One is postulate or hypothesis, i.e., propositions that are general but have no antecedent. A postulate is a belief, requiring commitment, and draws on the world of value.
Another domain is facts, i.e., propositions that are particular and have an antecedent. Facts are implied by and require the physical world.
Lastly, there are propositions, such as first cause, that are particular and have no antecedent. The clearest example of such would be the unpredictable "probabilistic" changes of state within the atom. At the human level, events whose antecedents are not sufficient to account for the effect, such as intuition, invention, mutation of genes, etc., are all examples of this domain. I would add prime numbers but will not attempt to prove it.
Now, I do not myself consider the "dignity" of logic to be of a higher or even equal dignity to mathematics. Nevertheless the fact that it was at one time considered possible to logicize mathematies (and the Principia Mathematica 1925-27 of Russell was directed to that end), and the fact that there has been no official statement that mathematics cannot be logicized, puts mathematics under obligation to state its reaction to logic (either to exclude it or to include it).
Clearly the situation requires that mathematics recognize logic as one element, but any problem involving logic must necessarily include purpose, hypothesis and fact in addition to logic - a logical proof would be useless if it did not have a purpose, make a hypothesis and end with a conclusion to be confirmed by fact. The four domains thus introduced - logic, hypothesis, purpose and fact - will be found to correspond with the four levels already discussed.
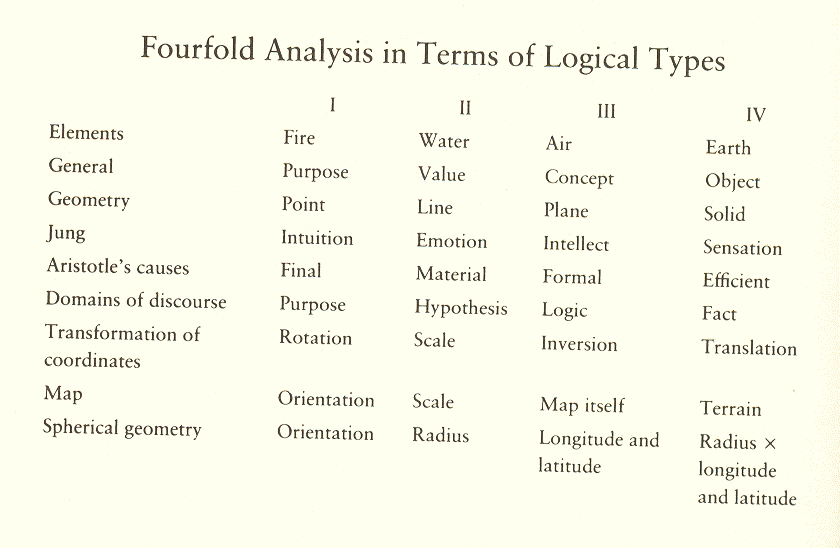
In the following chart, try, by reading the words in one column, to discern the common element in that column and its relation to the common elements of the other columns. Then observe how the four elements of each row
represent mutually independent and necessary aspects of any subject or situation. Be aware that words alone are often ambiguous. Thus, "object" can mean purpose. "Mind" can mean purpose, or obey, or opinion, or control. "Matter" can mean physical substance or value. "Substance" can mean almost anything. The listing of words in columns, each column representing a different domain or logical type, is essentially a formal device to assign meaning less ambiguously than common usage permits.
Let me close with a suggestion to indicate how the greater scope of mathematics can deal with what in logic is regarded as a paradox. The Cretan paradox (see p. 85) was disposed of by Russell with his distinction between logical types, which ruled that a statement cannot apply to itself.
Logic is two-valued; but it confuses the dichotomy true versus false with the dichotomy consistent versus inconsistent. In the case of the Cretan paradox both dichotomies are involved. It is because of the issue of consistency that the statement, "All Cretans are liars," when uttered by a Cretan, is a paradox.
Mathematics encounters a similar difficulty in the expression x squared = -1, but resolves the problem by creating a second axis perpendicular to the plus-minus axis, the socalled imaginary axis. This imaginary axis, when interpreted as time, has application to a wide range of timedependent phenomena - oscillations - from the negative energy states of the atom and the nucleus to the periodic motion of the planets.
The two dichotomies, consistent-inconsistent and truefalse, can be placed on the four levels as follows: I) inconsistent, II) false, III) consistent, IV) true. (The merit of inconsistency is novelty, and the merit of fiction is that it is not true.) "Consistency," said Emerson, "is the hobgoblin of little minds."
Mathematics, Physics & Reality